Two-Column Proof in Geometry (Definition & Examples)
Two-column proof definition
Two-column proof in geometry is only one of three ways to demonstrate the truth of some mathematical statement. Yet it is one of the most reliable methods, since it compels the geometrician, or at least the geometry student, to back up every claim with real evidence.
Among the many methods available to mathematicians are proofs, or logical arguments that begin with a premise and arrive at a conclusion by delineating facts.
Writing a proof is a challenge because you have to make every piece fit in its correct order. Most geometry works around three types of proof:
Paragraph proof
Flowchart proof
Two-column proof
Paragraphs and flowcharts can lay out the various steps well enough, but for purity and clarity, nothing beats a two-column proof.
A two-column proof uses a table to present a logical argument and assigns each column to do one job, and then the two columns work in lock-step to take a reader from premise to conclusion.
Structure in two-column proofs
A paragraph proof tells a story, with each fact and reason laid out in a time order. That means you have to be extremely organized and possibly rewrite the paragraph multiple times before getting it right. A flowchart proof can be hard to follow, but at least it separates the mathematics from the reasoning in a clear way.
Only a two-column proof explicitly places the mathematics on one side (the first column) and the reasoning on the other side (the second or right column). As long as you keep the two sides lined up, you cannot fail to move the reader from one premise to the next, and finally to the conclusion.
The structure of a two-column proof must follow four basic precepts:

The first or left column has only mathematical statements, like "quadrilateral PINK is a parallelogram" or "side PI = side NK."
The second or right column has only reasons supporting the validity of those mathematical statements, like "Given," or "If the opposite sides in a quadrilateral are the same length, then the figure is a parallelogram."
Every logical, ordered step is numbered in both columns, so Step One on the left is supported by Step One on the right.
You end when you have proved your concept.
How to solve two-column proofs
A two-column proof is only a structure, like a skeleton. You must have five tools handy to work your way from premise to conclusion and complete the two column proof:

Givens – State what is given to you and the reader in the diagram or setup of the problem
A diagram – The diagram will clarify what the geometric figure is; if no diagram is provided, draw one!
Foundational knowledge – You must have a deep understanding of theorems and postulates so you can apply them quickly and logically; without understanding definitions, vocabulary, and relationships among geometric figures, you cannot move from argument to argument
Reasoning and thinking skills – This is no royal road; you may start out, run into a mental wall, and have to start again; thinking logically is a learned and difficult skill, so be patient and give yourself thinking time
Order – Two-column proofs proceed from one idea to the next in a logical, clear and concise way, reaching a conclusion and then stopping
How to write two-column proofs
You can write a two-column proof by drawing a horizontal line at the top of a sheet of paper and a vertical line down the middle. Label the left side "Statement" and the right side "Reason." Say you are asked to prove the Isosceles Triangle Theorem, which states that if two sides of a triangle are congruent, their opposite angles are congruent.
You will be given some information, like △WHZ has Side HW ≅ Side HZ, making it an isosceles triangle.
You are asked to prove ∠W ≅ ∠ Z.
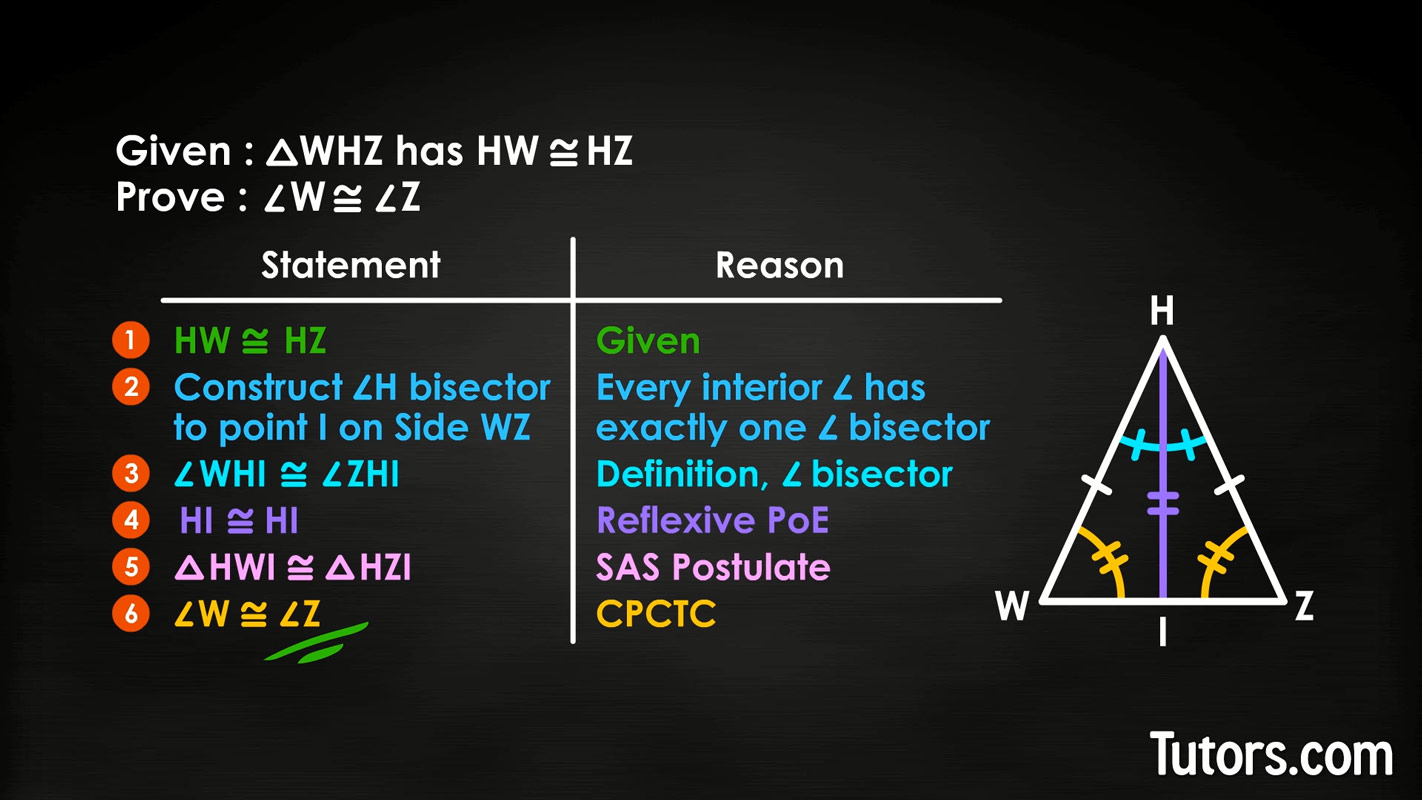
| Statements | Reasons |
|---|---|
| HW ≅ HZ | Given |
| Construct ∠H bisector to point I on Side WZ | Every interior ∠ has exactly one ∠ bisector |
| ∠WHI ≅ ∠ZHI | Definition, ∠ bisector |
| HI ≅ HI | Reflexive Property of Equality |
| △HWI ≅ △ HZI | Side-Angle-Side Postulate |
| ∠W ≅ ∠ Z | Corresponding parts of congruent triangles are congruent (CPCTC) |
This was a five-step proof. Most geometry proofs can be done in fewer than 10 steps. If you find yourself going past, say, seven or eight steps, you may be going down an inefficient or wrong path. How can you help yourself?
Two-column proofs & reasoning
One strategy for working through a two-column proof is to first consider the end: what is it you are asked to prove? Without considering a numbered item, put that down as the statement for the last position. Consider the reason for that statement; what would you need to prove that?
Another important detail is to draw a diagram or picture that exactly matches the given information. See what else is revealed by the given information, like complementary or supplementary angles, right angles you may not have noticed, or equalities of angles or sides.
Keep your reasons handy, especially if you have not memorized a huge collection of axioms and theorems. These can be postulates, other theorems, definitions, or properties. Remember properties can be from beyond geometry, too, like properties of equality.
Be willing to rearrange order. Do not get so attached to your proof that you persist in trying to "make it work" if it has led you down a blind path.
Start simple. If you are struggling with a complex proof of a tough problem, see if you can find smaller, more easily provable chunks within it.
The surest way to get better at two-column proofs is to practice writing them.
Showing off
Once you are really confident in your ability to write two-column proofs, you can show off a bit by writing Q.E.D. at the end, which is the abbreviation for the Latin phrase, quod erat demonstrandum, "that which has been demonstrated." It signals to your mathematics teacher and others that you are finished, you have completed the proof.
Lesson summary
Now that you went through both columns from top to bottom of this piece, you are able to understand and appreciate the value of proofs in mathematical reasoning. You can also recognize and name the three kinds of mathematical proof, identify the elements of two-column proofs, and even write your own two-column proof.