Collinear Points in Geometry (Definition & Examples)
Collinear points definition
Mathematicians use words very exactly. In Euclidean geometry, Collinear points are points that all lie in the same line, whether they are close together, far apart, or form a ray, line segment, or line.
col means "together." Our word college comes from the same prefix. Linear means line. The combination, collinear, means points together on a single line.
Collinear points in real life
Anytime you have a series of individual items in a single straight line, you have models of collinear points. Suppose you have eggs in a carton; each egg in one row is a collinear point:
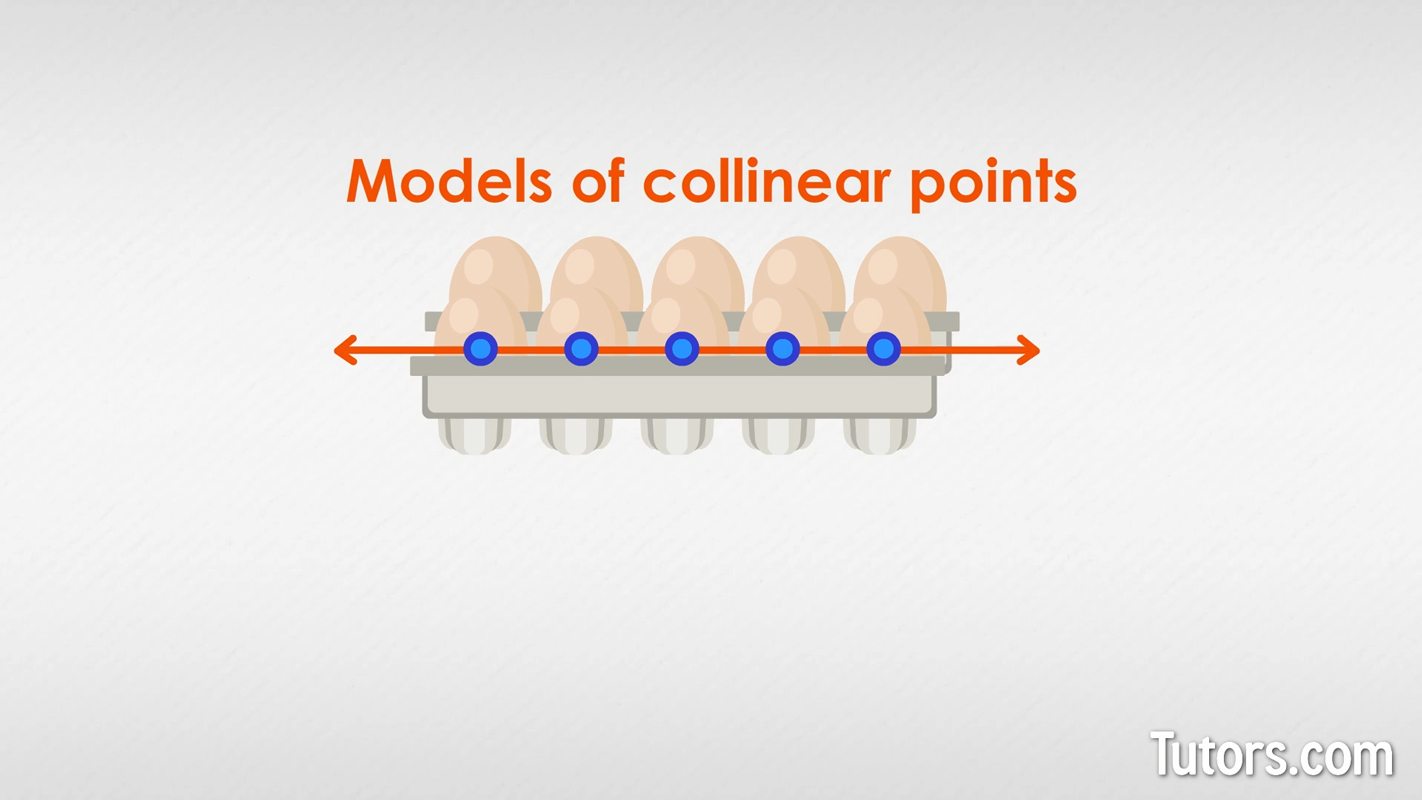
Students seated at a long cafeteria table are collinear. Football players on the line of scrimmage are collinear. Rings on a shower curtain, plants in one row in a garden, numbers on a ruler, moviegoers in a ticket line, and commuters seated on a train are collinear.
For real-life examples to be good models of collinear points, you need to be able to draw a straight line through them. Think of the individual kernels on one row of an ear of corn.
Non-collinear points
What is not a model of collinear points? The angle marks around the curved edge of a protractor, for one thing. Neither are spirals, helixes, all five corners of a pentagon, or points on a globe.
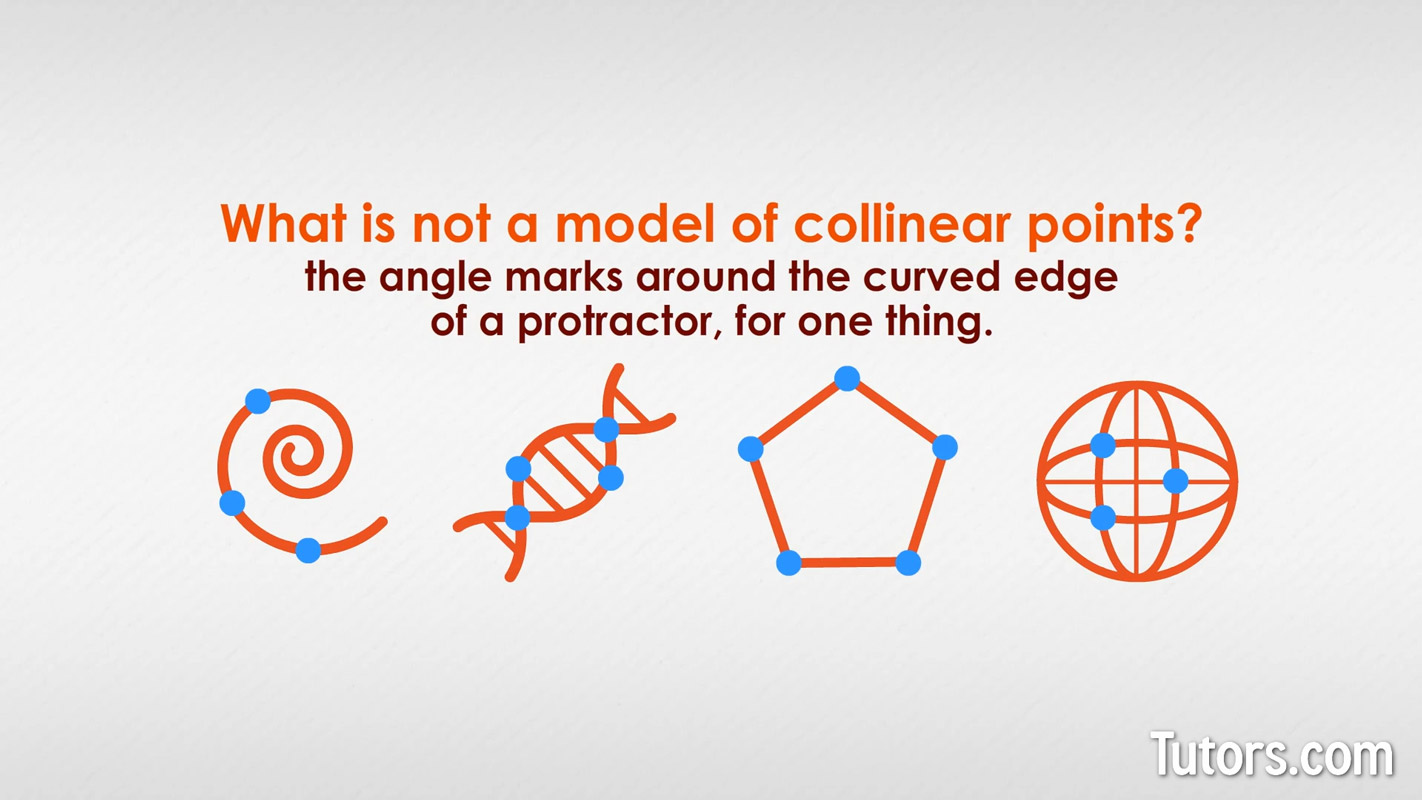
Non-collinear points are a set of points that do not lie on the same line. Picture a sushi roll in front of you. A second skewer of food sitting next to ours would not have any points collinear with our skewer, since they are all on a different skewer or line.
Points must lie on the same line to have collinearity. If picture a right triangle with two points label on two different sides points L and R. If point L on the hypotenuse and point R on the base, then point L and point R are non-collinear
Collinear points in geometry
Very often, collinear points appear in geometric figures such as quadrilaterals, triangles, parallelograms, and more. Take this kite with two diagonals intersecting at Point S:
Two sets of collinear points appear around the diagonals in this geometric figure:
K−S−T
I−S−E
But you can also find all these other collinear points since only two points determine a line:
KS
ST
IS
SE
KI
IT
TE
EK
Collinear points examples
We will leave you with a side view of a little street brazier for making skewered meat kebabs. Notice the legs cross and have a bottom brace, which creates two triangles to keep the brazier stable. Can you find at least 10 sets of collinear points?
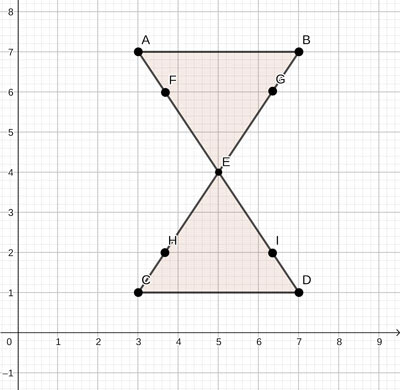
We are sure you saw sets like points A and B, C, and D, and points A−F−E−I−D, but did you also pick up on ones like CH, HE, EG, and GB?
The points C−H−E and E−I−D, which form two sides of a triangle (the bottom triangle) are also collinear.
Look at points H−E−G and E−G−B. Each of these three points are collinear as well. Keep looking; more sets of collinear points are waiting to be found!
Coplanar points
We now know that collinear points, sometimes spelled "colinear" (just on L), are points that lie on a straight line. But what about coplanar points? In a three dimensional world, coplanar points are a set of points that lie on the same plane. Learn more about coplanar points.