Cardioid - Definition, Equation, Graph & Examples
Cardioid definition
A cardioid (from Greek, "heart-shaped") is a mathematically generated shape resembling a valentine heart or half an apple. Constructing a cardioid on a polar graph is done using equations.
Imagine if you had a circle of a given radius and you rotate another circle of equal radius around it. Fix a point on the rolling circle and trace that point's path as the circle rolls around the circumference of the stationary one. The path that point traces is a cardioid.
Nature has already performed this feat with many apples. With an apple seated on its bottom, a single halving cut reveals the two cardioid shapes of each half.
Nature finds the cardioid very handy, using it also in leaves of the Galax plant, Frogbit (Hydrocharis morsus-ranae), and eastern sowbread (Cyclamen coum).
Cardioid equation
For drawing cardioids on graph paper, the best format is polar form. Rather than right-angle, rectangular x-value and y-value coordinates, a polar form uses polar coordinates.
To review, polar coordinates are expressed not as (x, y) but as (r, θ) where r is the distance of a line segment with one endpoint at the point of origin, O. The angle, θ, is a measure of the central angle created between the polar axis, x, and our line segment. That angle is always measured counterclockwise.
The polar axis is traditionally oriented to be horizontal on the right side of the polar graph, so our angle θ rises up from it on the right side of the graph, too.
To make measurement easier, you can choose any number of concentric distance rings from O, or you can leave the rings off entirely. You can also choose between measuring θ in degrees or, more usually, radians.
Horizontal cardioid equation
The polar form of an equation that will yield a cardioid has variables of r and θ. Points will be in (r, θ) format.
Since θ can be any angle, the resulting cardioid can orient horizontally or vertically. Let's use a circle with a labelled radius aa (we use aa so we do not confuse radius with our other variable, r, the distance from Origin, O).
To make a horizontal cardioid, the equation is:
Notice the ± that makes a left-facing and right-facing cardioid possible.
Vertical cardioid equation
To make a vertically oriented cardioid, the equation is:
This equation gives you both a right-side up and upside-down cardioid
Cardioid heart graph equation
Admittedly, cardioids generated by equations look far more like apple halves than valentine hearts.
Let's stop here to give away an equation for a flawless, vertical, valentine heart:
You can plug this into a graphing calculator, Google search, or online plotter and get a lovely little valentine.
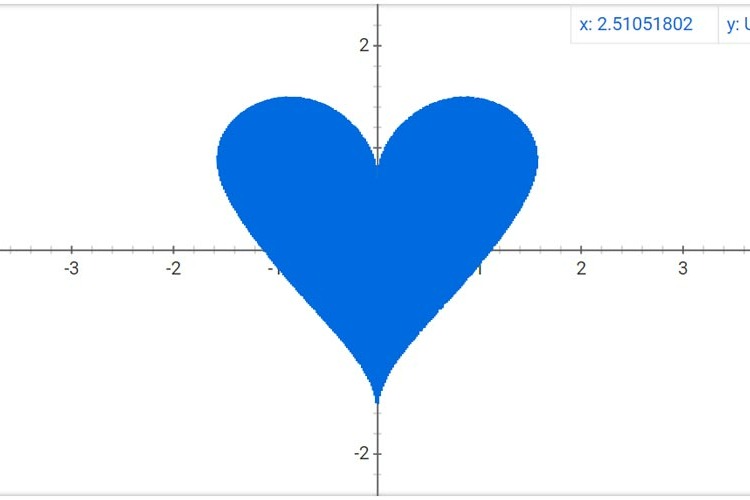
Cardioid Graph
Even before setting pencil to polar graph, you can learn something about polar equations and the shapes they may produce.
You have a few clues. First see which polar form has a cosine in it; that will be the horizontal cardioid; the sine function produces the vertical cardioid.
You can also determine the direction of the cardioid. For horizontal cardioids (using cosine), subtracting acos(θ) gives you a left-facing cardioid and adding acos(θ) points it to the right. For vertical cardioids (using sine), subtraction orients the cardioid upright; addition points it upside-down.
Next, notice that the radius of the circles producing the cardioids are also in the equations. That clue gives you a sense of how large or small your cardioids will be.
Cardioid graph examples
See what you can make of these:
Did you notice that examples 1 and 3 will produce horizontal cardioids? Examples 2 and 4 are vertical cardioids.
Which cardioid will be largest? Example 1 will be largest.
Which is smallest? Example 4 will be smallest.
Which cardioid will point to the right? Example 1.
Which points left? Example 3.
Which is the only right-side-up cardioid? Example 2.
For all four examples, the radius of the circles are the decimal values where a had been in our generic equations.