FOIL Method — How To FOIL & Examples

Written by

Malcolm McKinsey

January 12, 2023

Fact-checked by

Paul Mazzola

FOIL method

The FOIL Method is used to multiply binomials. FOIL is an acronym. The letters stand for First, Outside, Inside, and Last, referring to the order of multiplying terms. You multiply first terms, then outside terms, then inside terms, then last terms, and then combine like terms for your answer.

How to FOIL

The mnemonic FOIL tells us precisely what terms to multiply and in what order:

• First – multiply the first terms

• Outside – multiply the outside/outer terms

• Inside – multiply the inside/inner terms

• Last – multiply the last terms

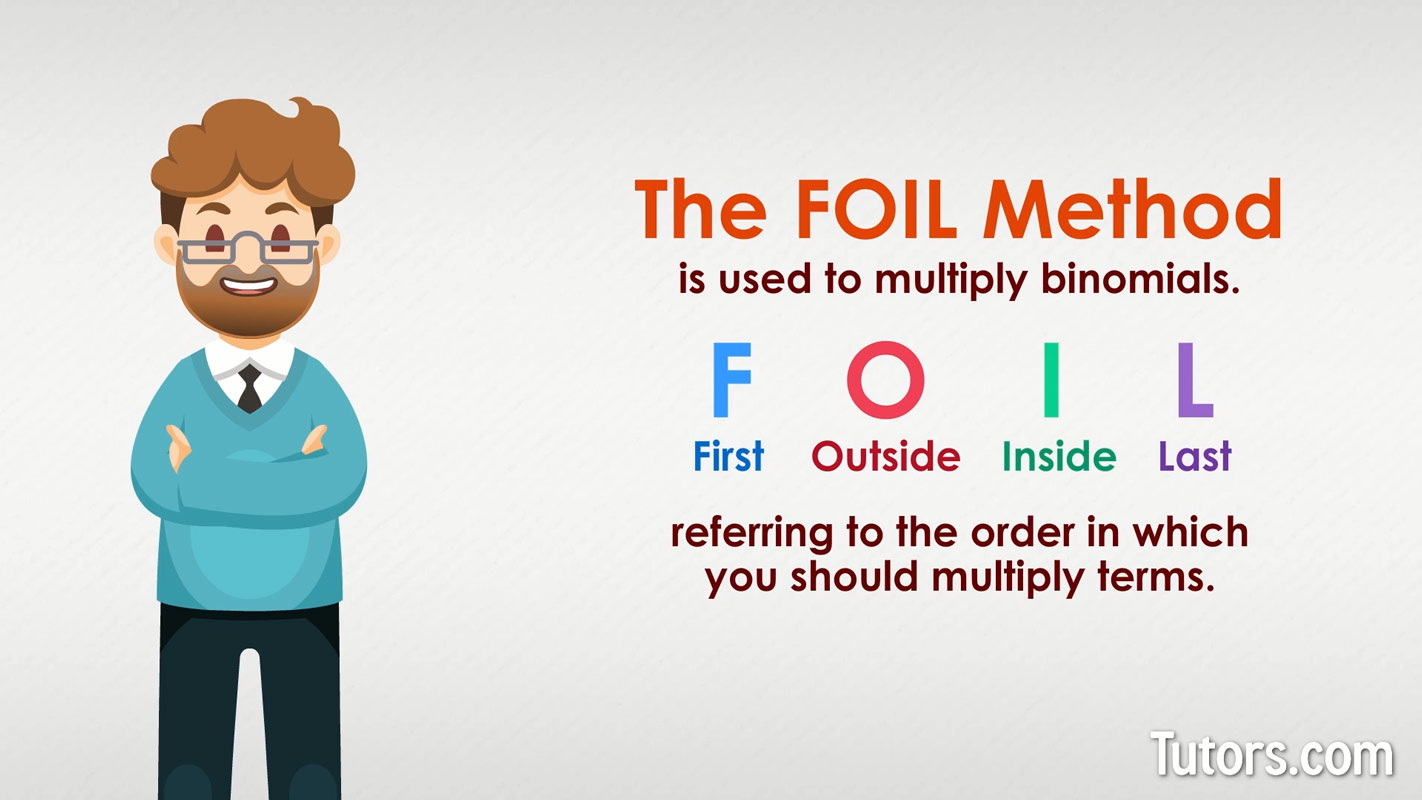
By following First, Outer, Inner, Last, we do not overlook any term in either binomial. All the terms in the first binomial are combined with the terms in the second binomial properly. Everything gets counted.

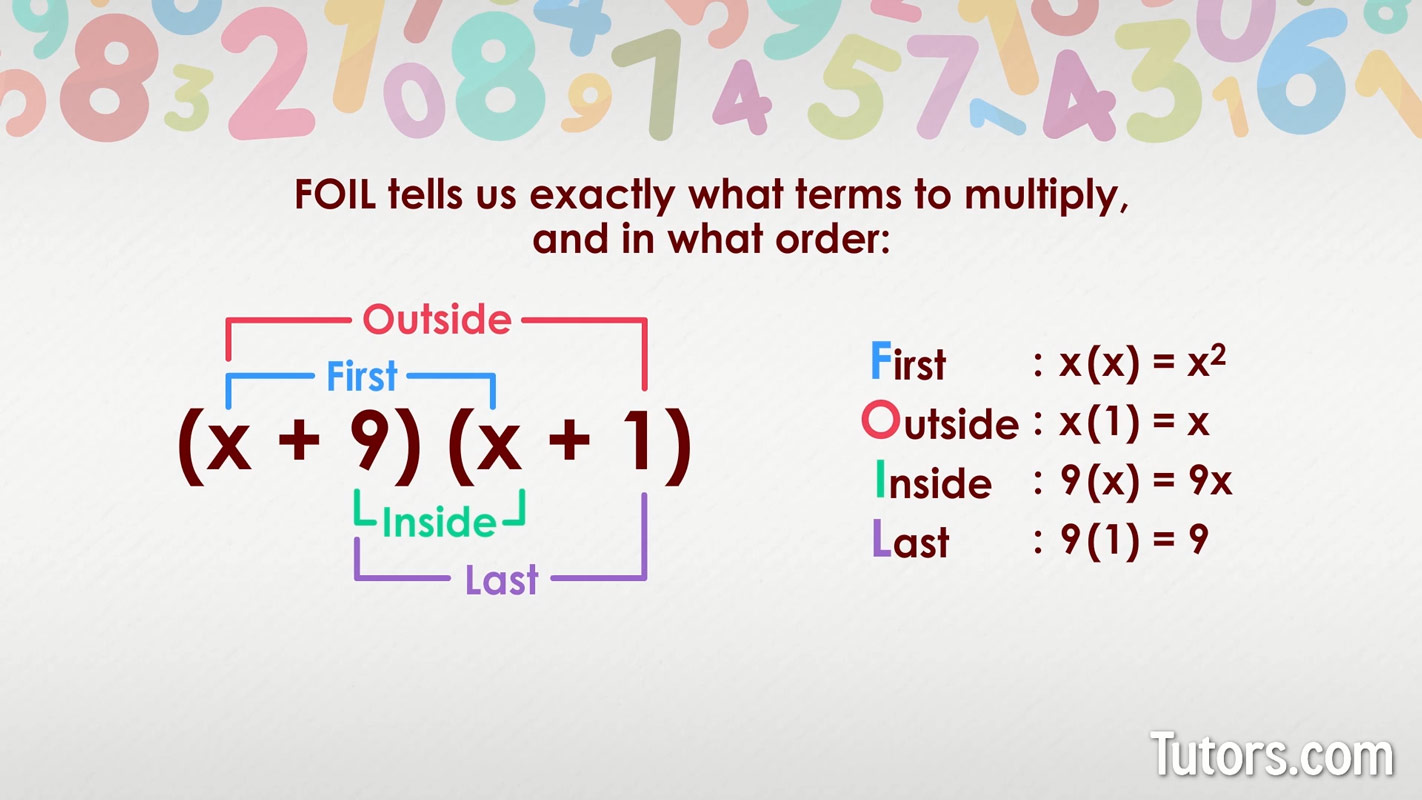
FOIL math examples

Let's apply the FOIL method to a couple of examples. Here we are multiplying two binomials:

Let's go through each step of FOIL to solve this multiplication problem:

1. First, multiply first terms of each binomial: $q\mathit{*}q={q}^{2}$

2. Outside terms are multiplied next: $q\ast (-7)=-7q$

3. Inside terms are multiplied next: $-3\ast q=-3q$

4. Last, multiply last terms of each binomial: $-3\ast \left(\mathit{-}\mathit{7}\right)=\mathbf{21}$

Put your four answers down on paper in the order you found them: ${q}^{2}-7q-3q+21$

Finish by combining like terms: ${\mathrm{q}}^{2}-10\mathrm{q}+21$

Now, let's use the FOIL method on this equation:

Go through each letter:

1. First – $5n\ast n=5{n}^{2}$

2. Outside – $5n\ast 6=30n$

3. Inside – $3\ast n=3n$

4. Last – $3\ast 6=18$

Put your answers together: $5{n}^{2}+30n+3n+18$

Combine like terms: $5{n}^{2}+33n+18$

Our final answer, the product of two binomials, contains three terms, so it is a trinomial.

Multiplying three binomials

Multiplying three binomials is a special case for FOIL because the FOIL method can only be used for multiplying two binomials at a time.

You can use FOIL to multiply three or more binomials if you pair them off, then factor the answer to the remaining binomial.

Here is a multiplication equation with three binomials:

To begin, we pair off the first two binomials:

Then, multiply them using the FOIL method, and we get:

Next, we combine like terms:

Now, we multiply our new binomial with the remaining binomial from the original equation:

Then, factoring and simplifying is the final step:

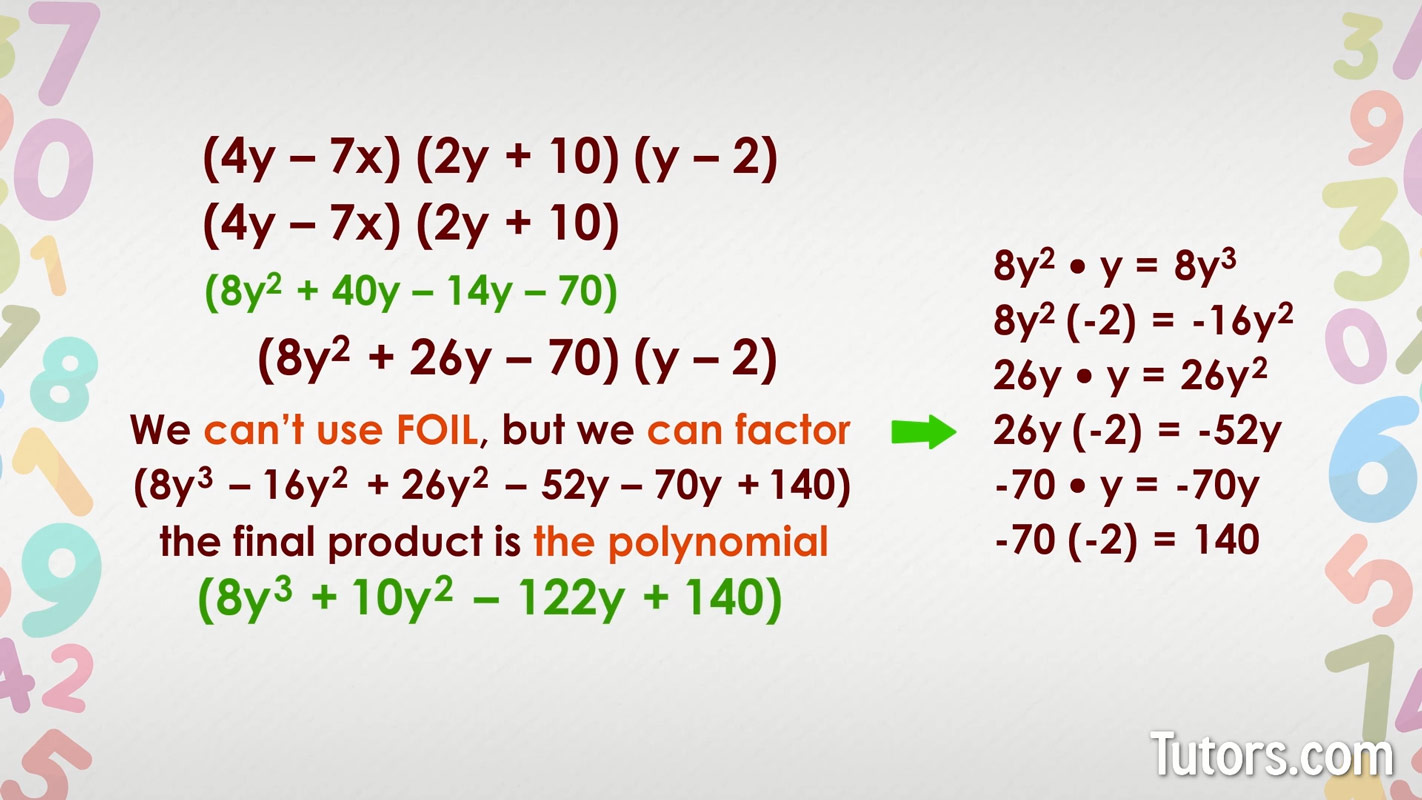
If you are faced with more multiplying two binomials, solve two at a time using FOIL until you are left with just one polynomial.

The term polynomial refers to an expression of constants, variables, and exponents that are added, subtracted, or multiplied, like the highlighted answer above. Each term on its own is called a monomial.