Congruent Figures — Definition, Shapes & Examples

Written by

Malcolm McKinsey

January 11, 2023

Fact-checked by

Paul Mazzola

What are congruent figures?

Congruent figures in geometry are identical in shape and size. Congruent figures have congruency. That seems simple enough, but congruent figures need not be turned the same way or face the same direction to be congruent.

Suppose you have two playing cards from two different decks, both Queens of Spades. Everything about these cards is the same:

• Size

• Shape

• Color

• Details

If we returned them to the decks, would we know which card was from which deck? No. The two cards are congruent, meaning they are identical in size and shape. The cards are rectangles with congruent sides and congruent interior angles.

In geometry, we do not worry about color; we pay attention only to size and shape. Suppose we turn one card on its side:

They are still congruent, even though one is rotated. Rotation does not interfere with congruency.

Congruent definition in geometry

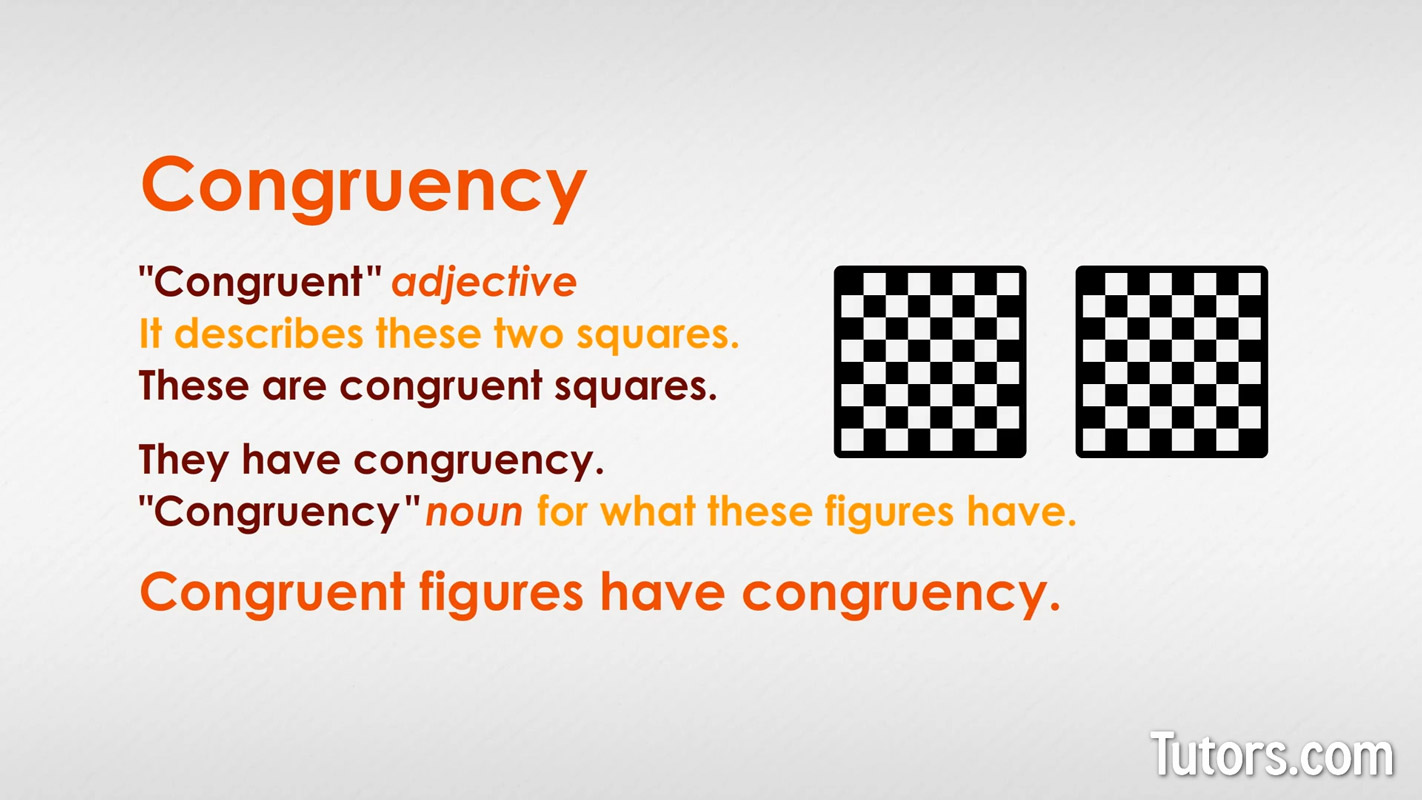
The word "congruent" is an adjective, and it describes two objects where their corresponding parts are identical. Congruent figures, like these two squares have congruency. Whether you have just two figures or a whole chessboard of congruent squares, they are all congruent.

Transformations in geometry

In transformation geometry, we can manipulate shapes in four ways:

1. Rotate (turn)

2. Translate (slide)

3. Reflect (flip)

4. Dilate (enlarge or shrink)

For the first three ways, congruent figures stay congruent. Rotate the Queen of Spades, and she is still the Queen. Slide the card around the table, and our Queen is still congruent with the other playing card.

Reflect a congruent figure (turn it to a mirror image), and it is still congruent.

Similar vs. congruent

If we enlarge or shrink the Queen, it is still the same shape, but they are now different sizes. The shapes still have congruent angles, but the line segments that make up the card are now different lengths, so the two shapes are no longer congruent. Dilating one of two congruent shapes creates similar figures, but it prevents congruency.

Figures are similar if they are the same shape; the ratios and length of their corresponding sides are equal. So, are congruent figures similar? Technically, yes, all congruent figures are also similar shapes. But not all similar shapes have congruency.

In geometry, similar triangles are important, and three theorems help mathematicians prove if triangles are similar or congruent.

• Side-Angle-Side (SAS)

• Side-Side-Side (SSS)

• Angle-Side-Angle (ASA)

Congruent shapes examples

Usually, we reserve congruence for two-dimensional figures, but three-dimensional figures, like our chess pieces, can be congruent, too. Think of all the pawns on a chessboard. They are all congruent. The geometric figures themselves do not matter. You could be working with congruent triangles, quadrilaterals, or even asymmetrical shapes. Here are two congruent figures with an example of one rotated, one translated up, and one reflected (flipped):

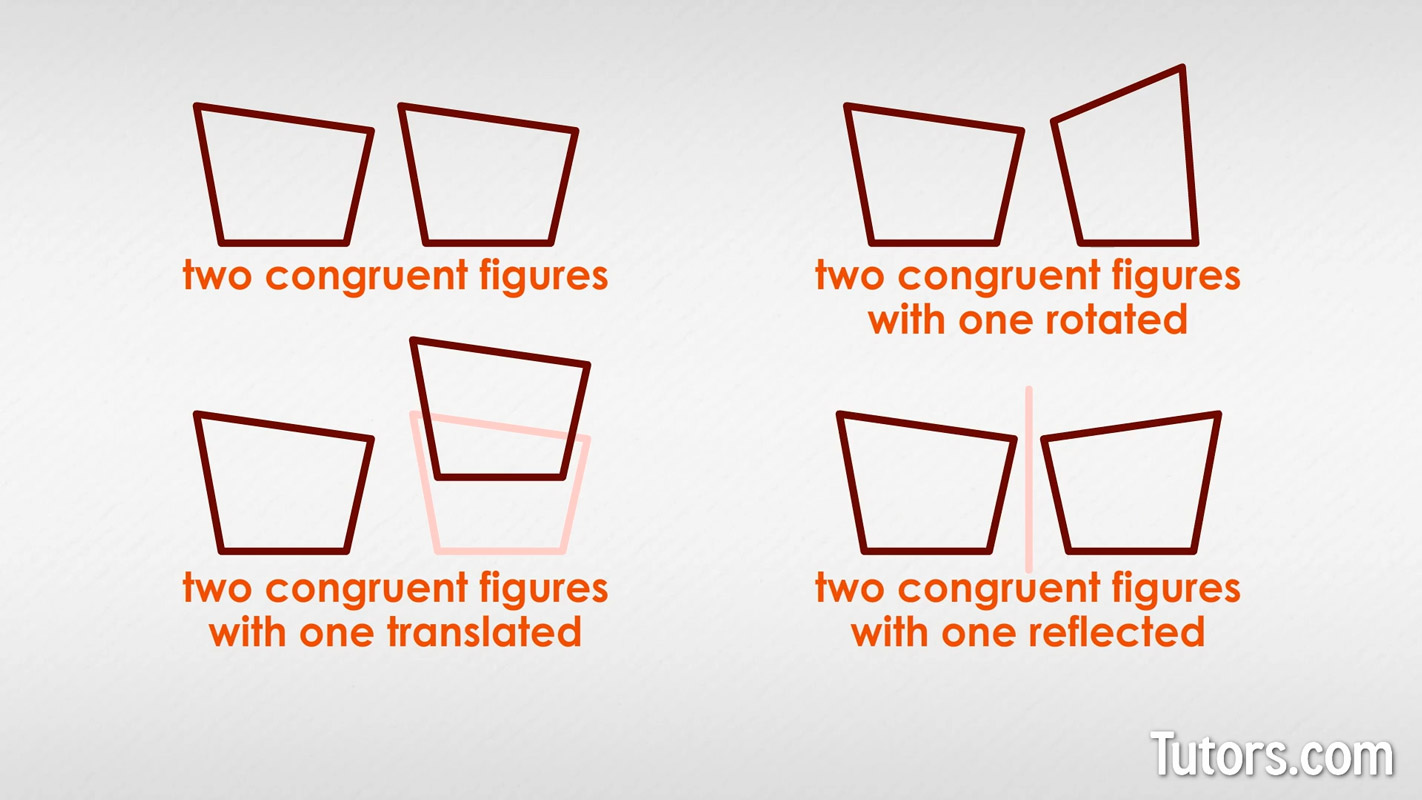
To summarize, congruent figures are identical in size and shape; the side lengths and angles are the same. They can be rotated, reflected, or translated, and still be congruent. They cannot be dilated (enlarged or shrunk) and be congruent.