Quadratic Formula — Equation, How To Use & Examples

Written by

Malcolm McKinsey

January 11, 2023

Fact-checked by

Paul Mazzola

Quadratic formula definition

The quadratic formula is an algebraic formula used to solve quadratic equations.

A word with “quad" in it usually implies four of something, like a quadrilateral. But the origin of the word "quadratic" means “to make square,” as in length times width (l x w).

In math, the meaning of square is an exponent to the second degree:

• ${x}^{2}$

• ${2}^{2}$

• ${b}^{2}$

So a quadratic polynomial has as its highest value something to the second degree; something squared.

Quadratic equation in standard form

The quadratic formula is used to solve quadratic equations. Consider a quadratic equation in standard form:

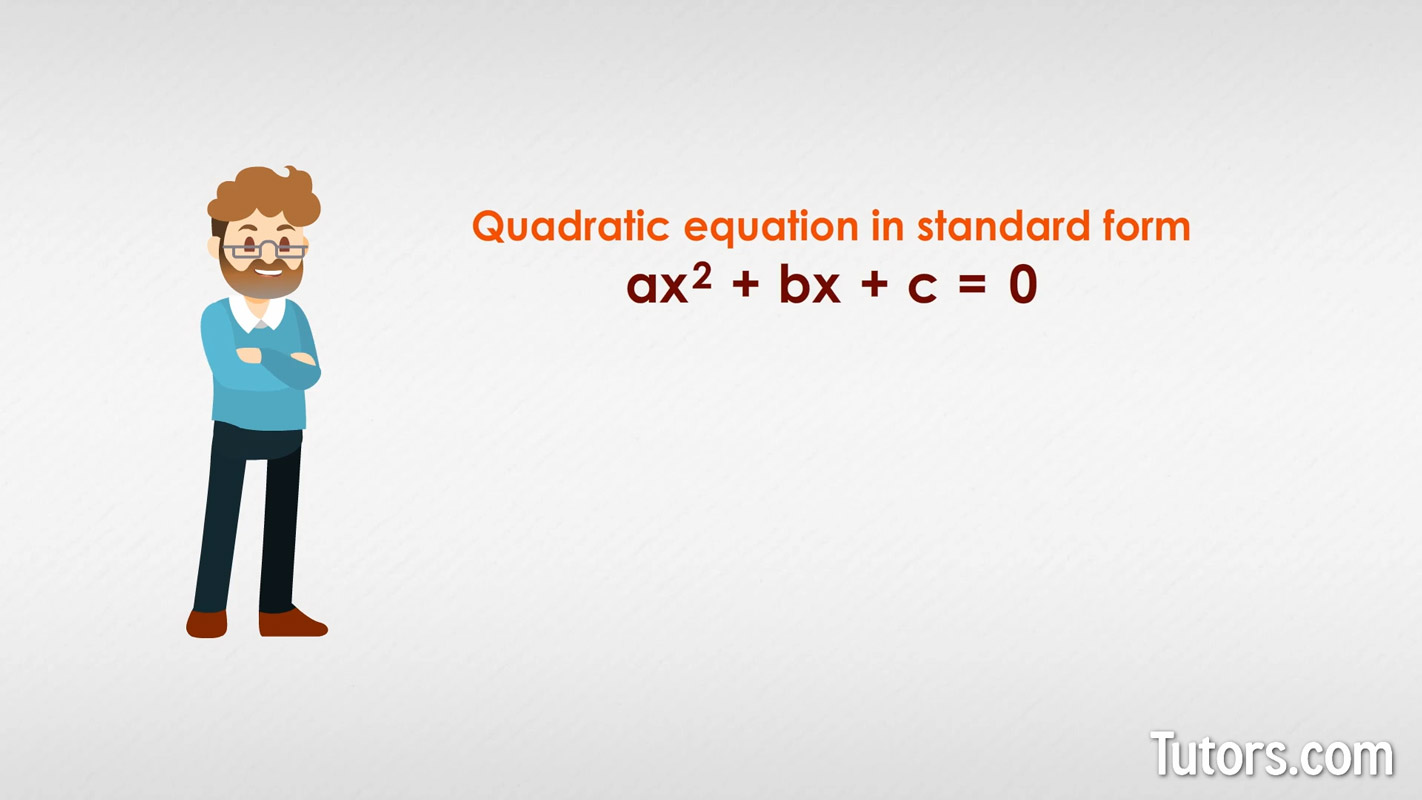
You may also see the standard form called a general quadratic equation, or the general form. So long as a ≠ 0, you should be able to factor the quadratic equation. Sometimes, though, this gets confusing or messy, or you cannot factor it.

What is the quadratic formula?

You can always find the solutions of any quadratic equation using the quadratic formula. The quadratic formula is:

You can use this formula to solve quadratic equations. Or, if your equation factored, then you can use the quadratic formula to test if your solutions of the quadratic equation are correct.

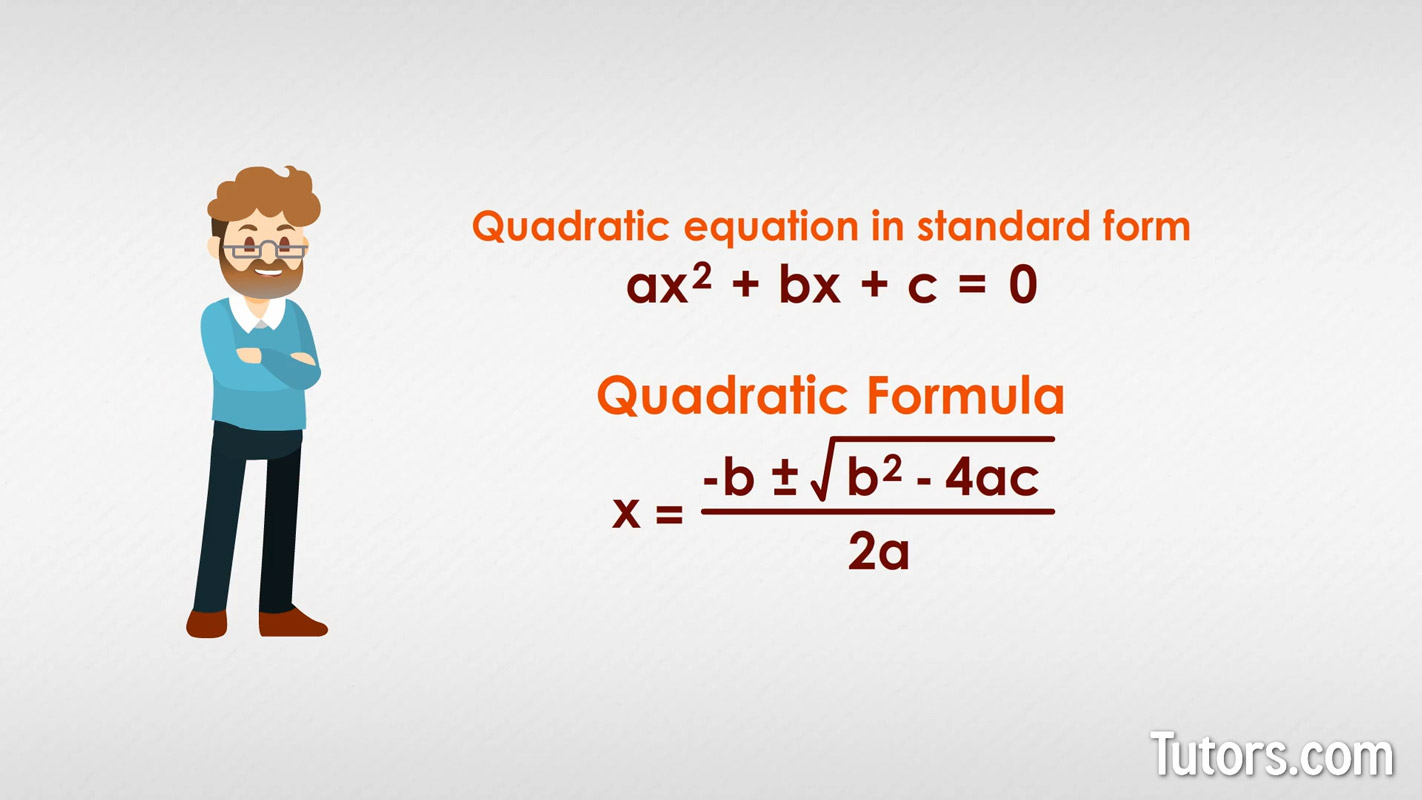
The expression ${b}^{2}-4ac$, which is under the √ (sqrt) inside the quadratic formula is called the discriminant. The discriminant is used to determine how many solutions the quadratic equation has.

• if ${b}^{2}-4ac=0\to$ 1 solution

• if ${b}^{2}-4ac>0\to$ 2 solutions

• if ${b}^{2}-4ac<0\to$ no real solution

How to use the quadratic formula

Let's start with an easy quadratic equation:

For the quadratic formula to apply, the equation you are untangling needs to be in the form that puts all variables on one side of the equals sign and 0 on the other:

Solving quadratic equations steps

Our quadratic equation will factor, so it is a great place to start. First we factor the equation. We are seeking two numbers that multiply to 6 and add to 5:

We can see that either expression equals 0 (since multiplying it times the other expression yields 0). We can set each expression equal to 0 and then solve for x:

Our two values for x are -2 and -3.

Comparing our example, ${x}^{2}+5x+6=0$, to the standard form of the quadratic equation (which can also just be called “the quadratic”), we get these values:

• a=1

• b=5

• c=6

Now we can use those in the quadratic formula and check, since we already know our answers are -2 and -3:

The ever-reliable quadratic formula confirms the values of x as -2 and -3.

Find x-intercepts

In an equation like $a{x}^{2}+bx+c=y$, set y=0 and work out the equation. The possible x-values will be the x-intercepts; where you line crosses the x-axis.

Think of how much we know about our graph solution even before we perform any algebraic calculations:

• Since the equation will yield two solutions for x, we have two x-intercepts

• We will have a parabola

• We can start plotting the parabola with two ordered pairs, $({x}_{1},0)$ and $({x}_{2},0)$

• The vertex of the parabola will be between the two x-intercepts

By solving the algebraic equation, you have given yourself a head start on graphing the equation.

Graphing calculators will probably not be equal to the precision of the quadratic formula. Use the calculator to verify the rounded results, but expect them to be slightly different.

For example, suppose you have an answer from the quadratic formula with in it. Leave as is, rather than writing it as a decimal equivalent (3.16227766), for greater precision.

Quadratic formula examples

In solving quadratics, you help yourself by knowing multiple ways to solve any equation. Start solving a quadratic by seeing if it will factor (what two factors multiply to give c that will also sum to give b?).

You can also try completing the square. Are you still struggling? Then apply the quadratic formula.

Use any of these methods, and graphing, to check an answer derived using any other method. Use the quadratic formula to check factoring, for instance.

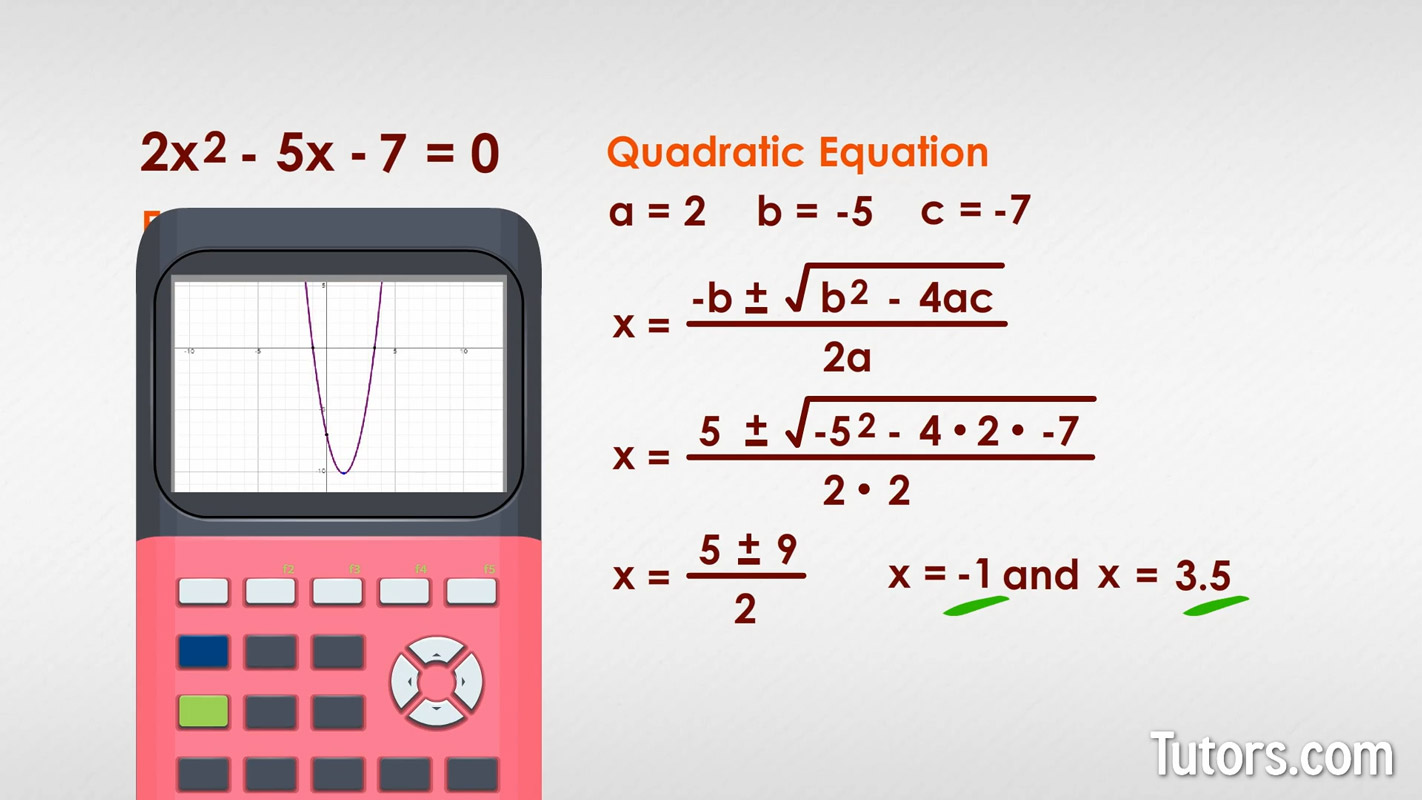
Let's try another example using the following equation:

First we can factor it:

Then we can check it with the quadratic formula, using these values:

• a=2

• b=-5

• c=-7

If you then plotted this quadratic function on a graphing calculator, your parabola would have a vertex of (1.25, −10.125) with x-intercepts of -1 and 3.5.

Here is a quadratic that will not factor: ${x}^{2}-7x-3=0$

No factors of -3 add to -7, so you cannot use factoring. What to do?

If all you knew was factoring, you would be stuck. But you know to try the quadratic formula, with these values:

• a=1

• b=-7

• c=-3

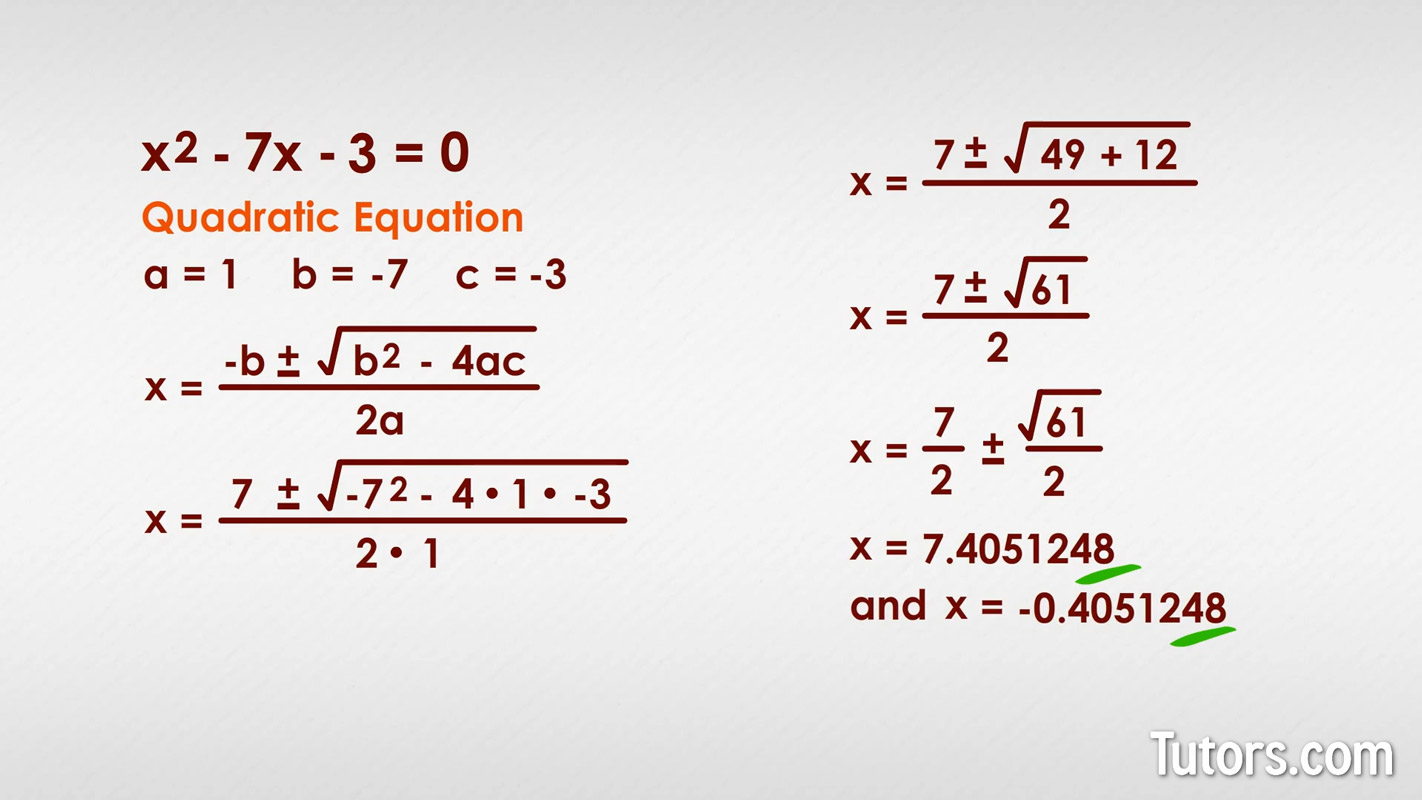
When to us the quadratic formula

Quadratic equations are actually used every day. They can be used to calculate areas, formulate the speed of an object, and even to determine a product's profit.

It is important that you know how to find solutions for quadratic equations using the quadratic formula.

When using the quadratic formula, you must be attentive to the smallest details. For example, placing the entire numerator over 2a is not optional. Everything, from -b to the square root, is over 2a.

Also, notice the ± sign before the square root, which reminds you to find two values for x.

That pesky bb right at the beginning is tricky, too, since the quadratic formula makes you use -b. Try not to think of -b as "negative b" but as the opposite of whatever value "b" is.

Suppose your b is positive; the opposite is negative. What if your original b is already negative?

Think: the negative of a negative is a positive; so -b is positive!

Under the square root bracket, you also must work with care. Sometimes ${b}^{2}$ is preceded by a negative sign, which means you are squaring all of b, even if it is negative. The square of a negative is a positive, so ${b}^{2}$ will always be a positive value.

The point: work very carefully. Keep track of your signs, work methodically, and skip nothing. Then use a different method to check your work.

No matter which method you use, the quadratic formula is available to you every time. It will always work.