Geometric Mean - How to Find, Formula, & Definition

Written by

Malcolm McKinsey

January 11, 2023

Fact-checked by

Paul Mazzola

Geometric mean definition

Geometric mean involves roots and multiplication, not addition and division. You get geometric mean by multiplying numbers together and then finding the ${n}^{th}$ root of the numbers such that the ${n}^{th}$ root is equal to the amount of numbers you multiplied. Geometric mean is useful in many circumstances, especially problems involving money.

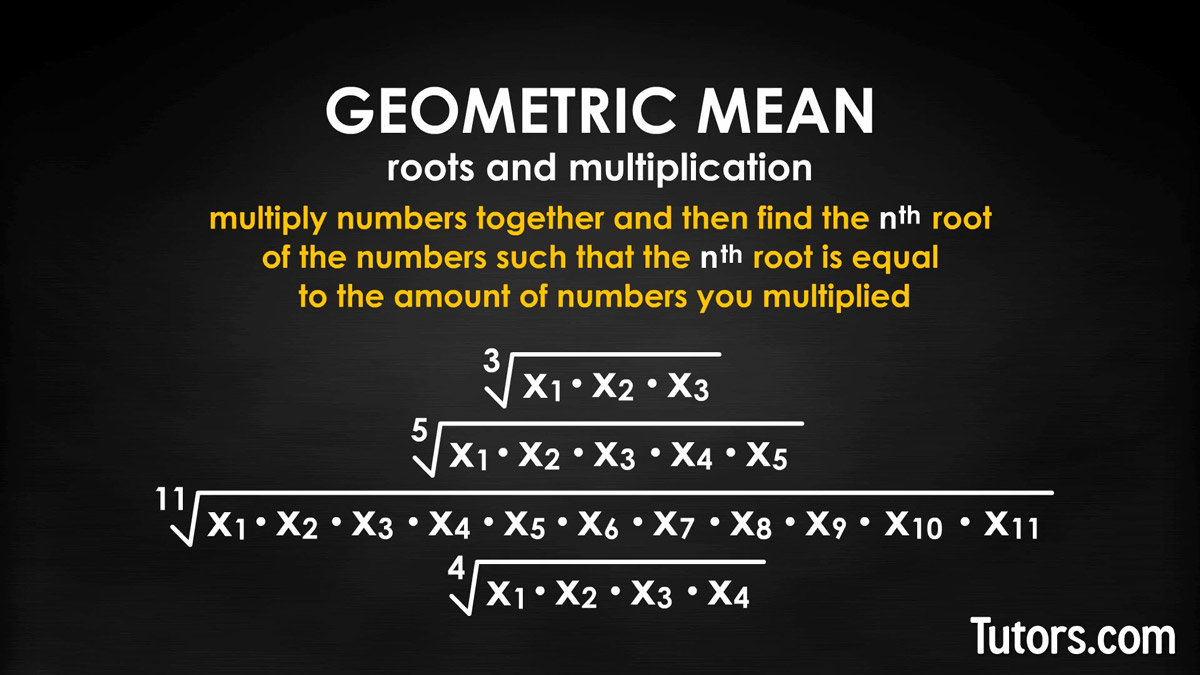
For example, if you multiply three numbers, the geometric mean is the third root of the product of those three numbers. The geometric mean of five numbers is the fifth root of their product.

Suppose we said we found the geometric mean using the 11th root of the numbers. That tells you that 11 numbers were multiplied together. To find the geometric mean of four numbers, what root would we take? The fourth root, of course.

Geometric mean vs. arithmetic mean

You are probably familiar with arithmetic mean, informally called the average of a group of numbers. You get arithmetic mean by arithmetic, or adding the numbers together and then dividing by the amount of numbers you were adding.

How to find the geometric mean

We will start with an easy example using only two numbers, 4 and 9. What is the geometric mean of 4 and 9?

Multiply 4 × 9. Then find the square root of their product (because you only multiplied two numbers):

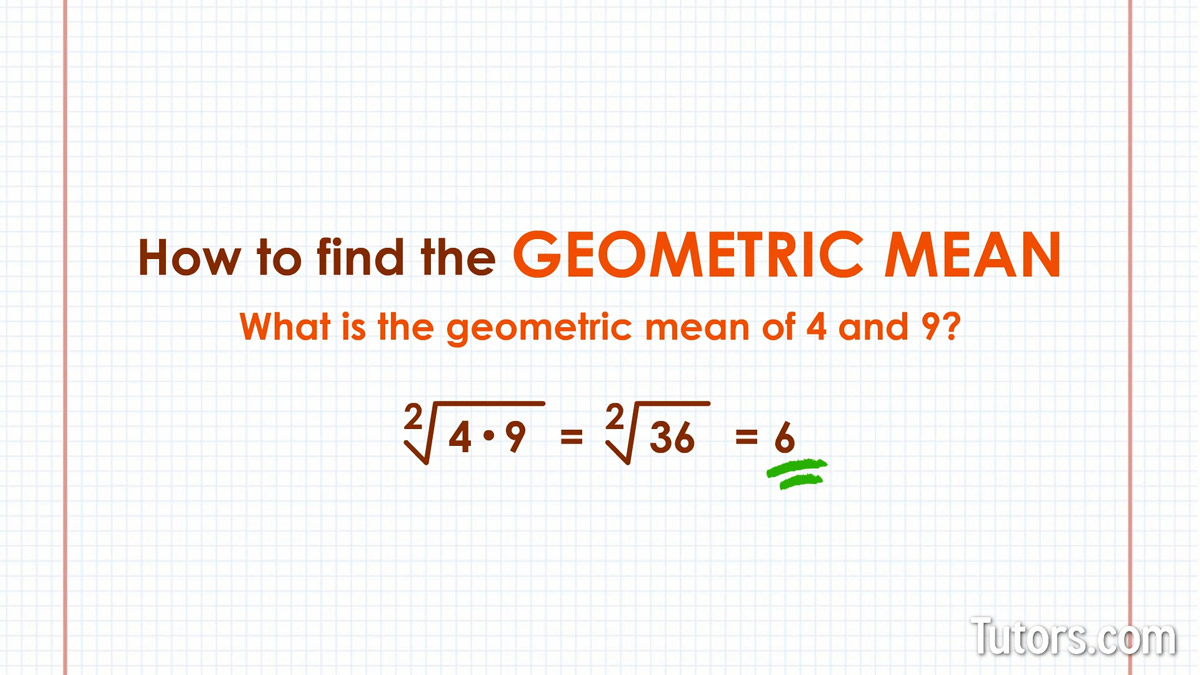
The geometric mean of 4 and 9 is 6.

Geometric mean formula

Let $n$ equal the number of terms we are multiplying, and let ${x}_{1}$, ${x}_{2}$, ${x}_{3}$ and so on up to ${x}_{n}$ be the different factors (the various terms).

Geometric mean theorem

This formula tells us to multiply all the terms (radicands) within the radical (the symbol for roots), and then to find the ${n}^{th}$ root of them where $n$ is how many radicands you have. You can separate whole number radicands with either an × or a * to show you are multiplying them.

Let's first try it with our earlier, easy example, and here the × is the symbol of multiplication:

We can substitute a * for the × to also show multiplication:

Now let's try a quick example with three terms:

The product of 3 x 6 x 12 = 216.

The cube root (the third root) of 216 is 6.

Our geometric mean is 6.

Uses for the geometric mean

Anytime we are trying to calculate average rates of growth where growth is determined by multiplication, not addition, we need the geometric mean. This connects geometric mean to economics, financial transactions between banks and countries, interest rates, and personal finances.

Your growth rate for money you have in bank deposits can be calculated using geometric mean, since your money grows at an advertised rate. You could not calculate this using arithmetic mean.

Geometric mean examples

The best way to become familiar with using the geometric mean is to use it. Use the formula to find the geometric mean of these six numbers:

Here is the formula again:

And here is the formula with our numbers:

The product of the radicands is found easily:

Now you must find the sixth root of 7,200:

The sixth root of $7,200=4.39429035137$. Our work is done!

Lesson summary

In this lesson we learned how to define the geometric mean, which is the ${n}^{th}$ root of a group of $n$ factors, how to find the geometric mean of any group of numbers by multiplying them and then taking the root equal to the total amount of numbers, how to apply the geometric mean to situations where growth rates are determined by multiplication, not addition, and how to write and use the formula for geometric mean.