20+ tutors near you & online ready to help.

TABLE OF CONTENTS

What is Surface Area? — Definition, Formulas & Examples

Written by

Malcolm McKinsey

January 16, 2023

Fact-checked by

Paul Mazzola

Dimensionality

Surface area of three-dimensional solids refers to the measured area, in square units, of all the surfaces of objects like cubes, spheres, prisms and pyramids.

One-dimensional figures have only one dimension, one direction that can be measured. A line is one dimensional, since it has only length but no width or height.

Two-dimensional figures have two dimensions: width and length. All plane figures are two dimensional or 2D. Think of a square, circle, triangle or rectangle.

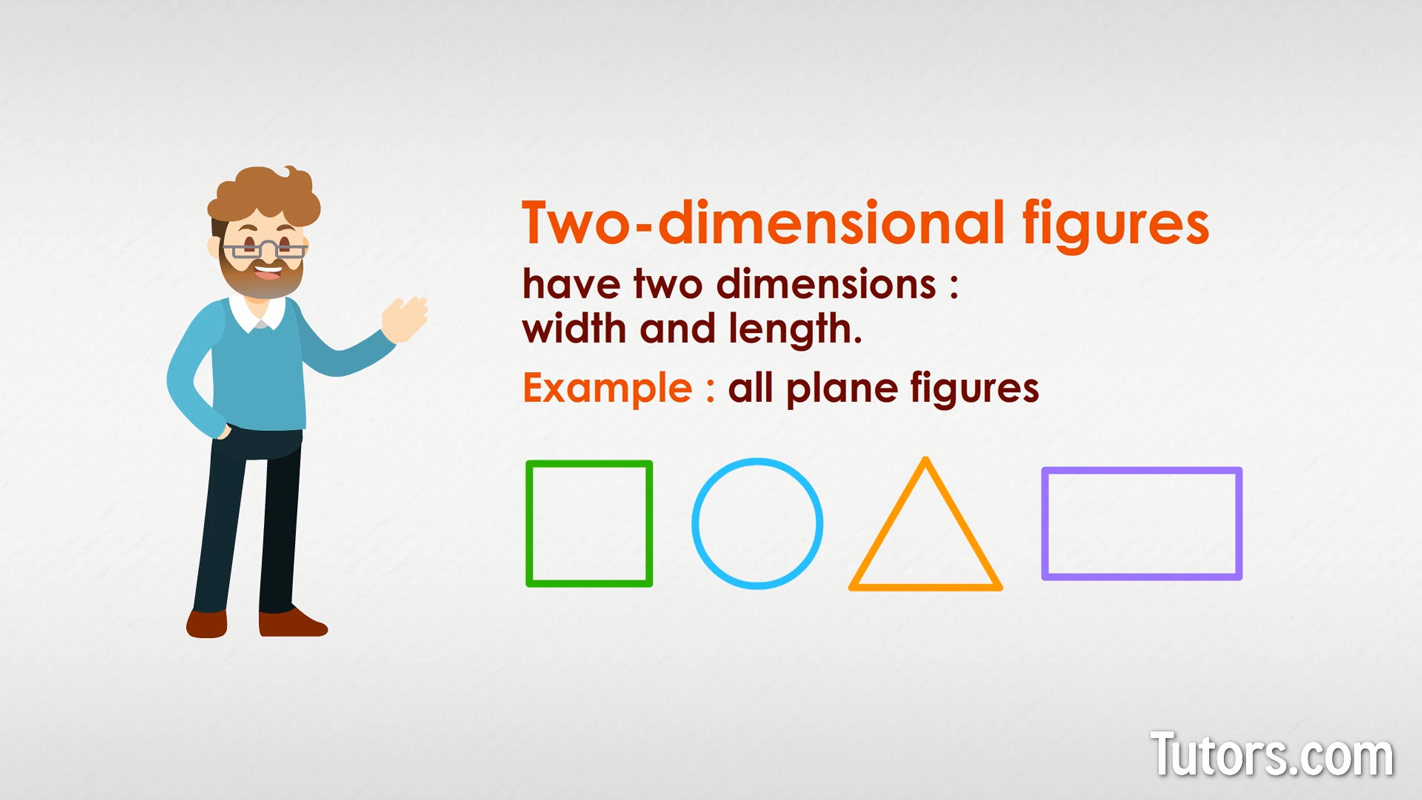
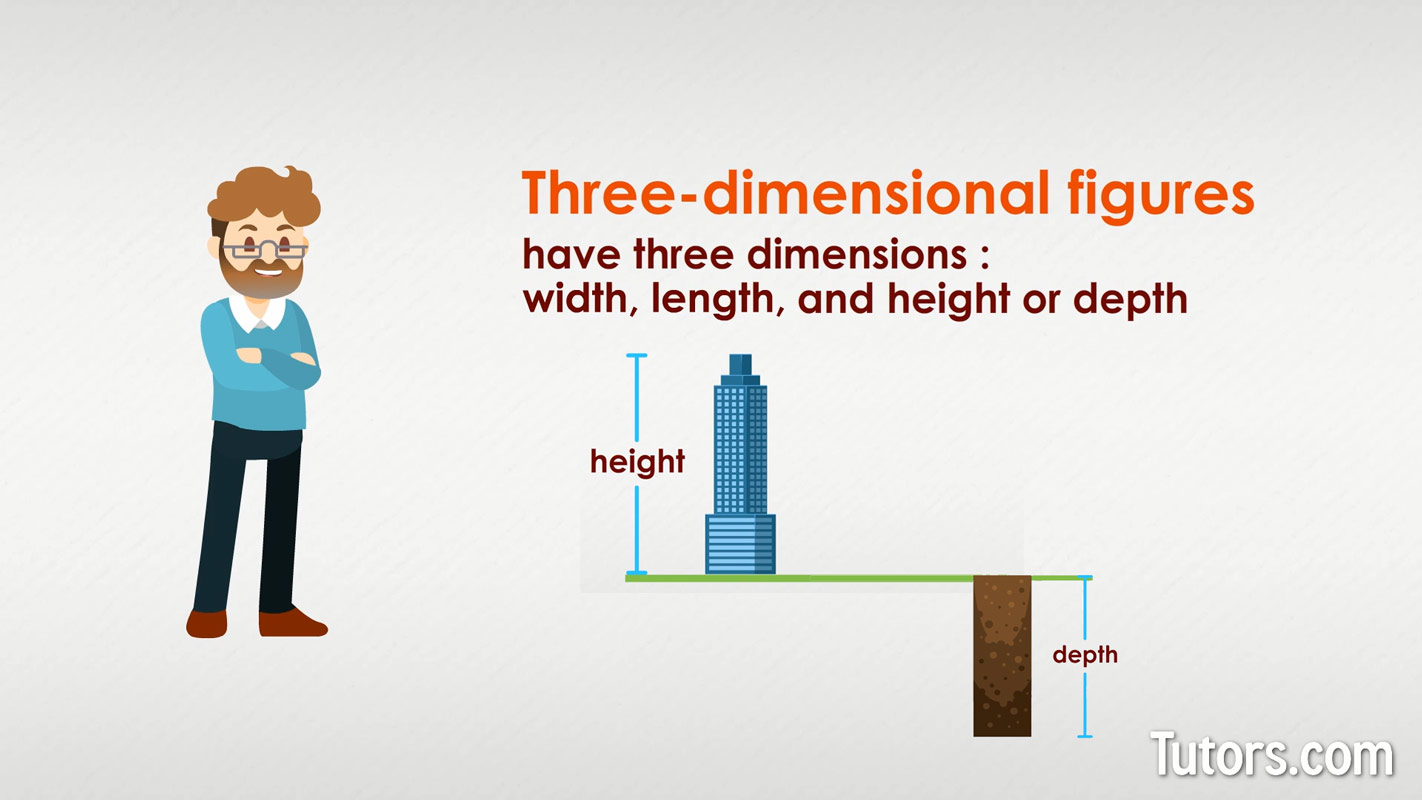
Three-dimensional figures have three dimensions: width, length, and height or depth. When dealing with 3D, we can use height or depth interchangeably, based on what is being measured.

We would use height to describe a skyscraper, but we probably would use depth to describe a hole in the ground. Three-dimensional solids include everyday objects like people, pets, houses, vehicles, cubes, cereal boxes, donuts, planets, shoe boxes, and mathematics textbooks. We live in a 3D world.

Get free estimates from geometry tutors near you.

Defining our terms

A 3D solid is a closed, three-dimensional shape. Examples of 3D solids are cubes, spheres, and pyramids.

A face of a 3D solid is a polygon bound by edges, which are the line segments formed where faces meet. Spheres have no faces.

A cube is a rectangular prism with six congruent, square faces.

A sphere is the set of all points in three dimensions that are equidistant from a given point. A hemisphere is one-half a sphere, its surface area including the circular cross section.

A prism is a 3D solid with two congruent, opposite faces (bases) with all other faces parallelograms of some sort. Examples of prisms are cubes and triangular, rectangular, hexagonal and octagonal prisms.

A right cylinder is a 3D solid with two circular, opposite faces (bases) and parallel sides connecting the circles. Any cross-section taken of a cylinder produces another circle congruent to the base.

A pyramid is a 3D solid with one polygon for a base (triangular, square, hexagonal - mathematically you have no limits) with all other faces being triangles. The Great Pyramid of Giza is a square pyramid.

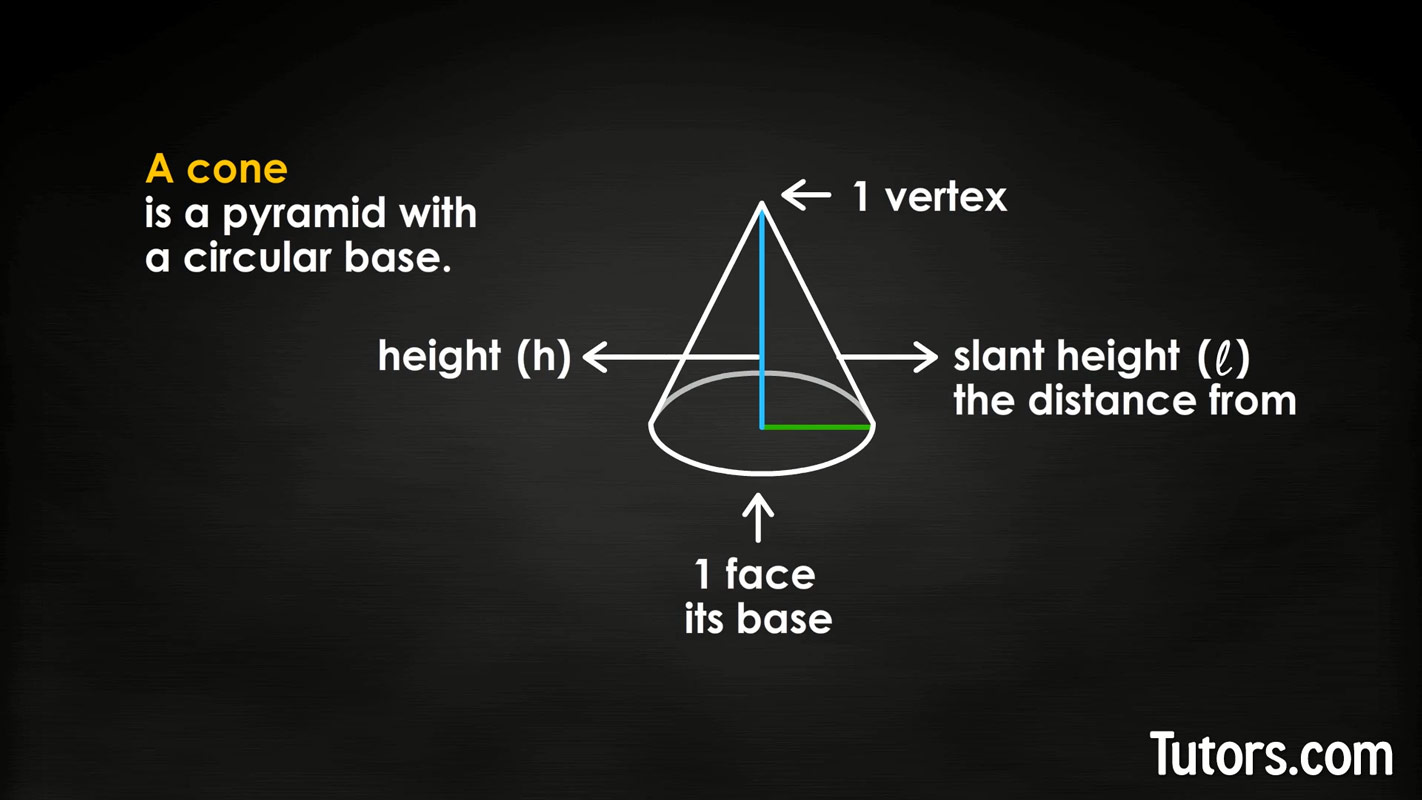
A cone is a pyramid with a circular base. A cone has only one face, its base, and one vertex. It has height, h, the perpendicular measure from base to vertex, and slant height, l, which is the distance from base to vertex along its lateral surface.

Surface area formulas for prisms

For every 3D solid, we can examine each face or surface and calculate its surface area. Then, adding all the individual surface areas, we can find the surface area of the entire solid.

Think of it as unfolding the 3D shape like a cardboard box. Lay out every face, measure each, and add them. This is what occurs with geometry nets.

Formulas work for all the prisms. The faces of prisms will be recognizable polygons, so let's review the area formulas for the basic polygons:

Try it!

Here is a triangular prism:

Total surface area of a triangular prism example

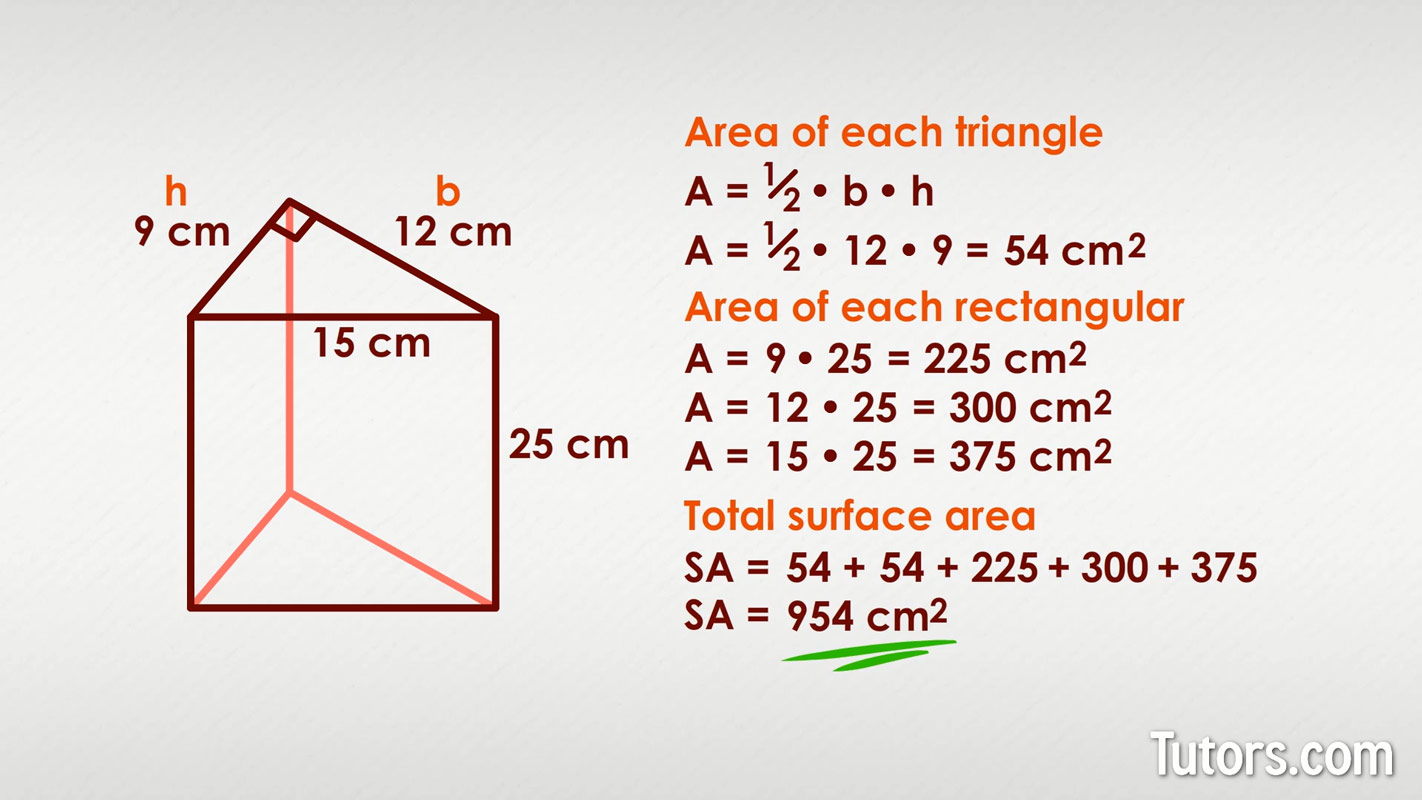
The area of each triangle is $\frac{1}{2}bh$ :

A=\frac{1}{2}(bh)

A=\frac{1}{2}(12cm\times 9cm)

A=\frac{1}{2}(108c{m}^{2})

A=54c{m}^{2}

Remember, though, we have two of these bases. Next, calculate the area of each of the three rectangular faces:

$9cm\times 25cm=225c{m}^{2}$
$12cm\times 25cm=300c{m}^{2}$
$15cm\times 25cm=375c{m}^{2}$

Then, add all the individual areas to find the total surface area:

SA=54c{m}^{2}+54c{m}^{2}+225c{m}^{2}+300c{m}^{2}+375c{m}^{2}=954c{m}^{2}

Square centimeters derive from the linear unit, centimeters. All surface areas of 3D solids are measured in square units, even when the objects are spheres, cylinders or cones.

Here is a rectangular prism:

Total surface area of a rectangular prism example

What is it's surface area?

Think: you need to measure three of the six faces, add them, and then multiply times 2, since the prism has three pairs of congruent faces. You must of course choose three dissimilar faces to capture length (l), width (w), and height (h):

SA=2\left(lw+lh+hw\right)

SA=2\left(21\text{'}\times 17\text{'}+21\text{'}\times 19\text{'}+19\text{'}\times 17\text{'}\right)

SA=2\left(21\text{'}\times 17\text{'}+21\text{'}\times 19\text{'}+19\text{'}\times 17\text{'}\right)

SA=2\left(\mathrm{1,079}f{t}^{2}\right)

SA=2\left(\mathrm{1,079}f{t}^{2}\right)

Here is a cube representing all the gold that has ever been mined on earth:

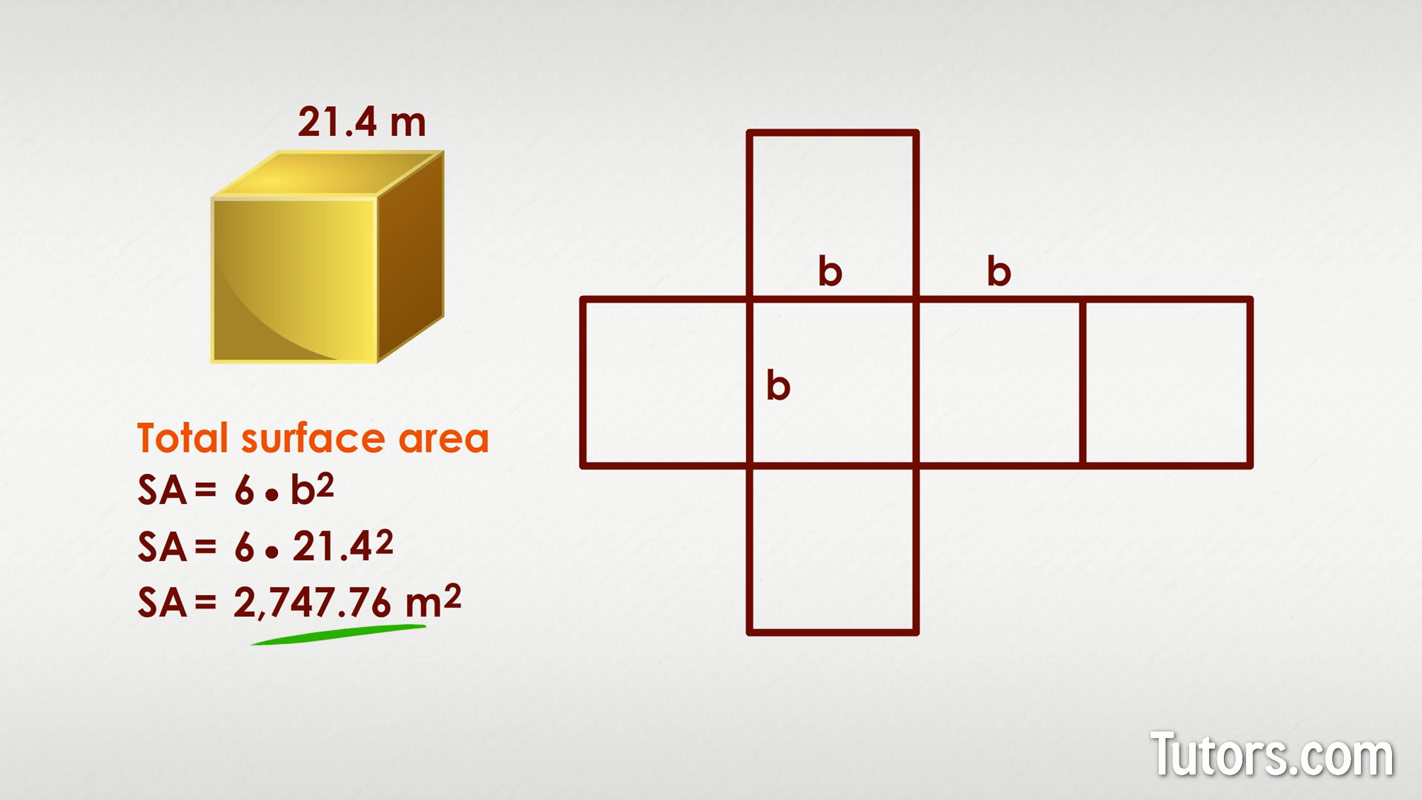
What is its surface area? Think: a cube is six squares, each with a length equal to width equal to height. For a cube with any side b, the formula is:

SA=6{b}^{2}

SA=6(21.4{m}^{2})

Get free estimates from geometry tutors near you.

SA=\mathrm{2,747.76}{m}^{2}

Surface area formulas for non-prisms

For pyramids, the surface area formula for a pyramid with a base area, A, perimeter of base, p, and slant height, l, is:

SA=A+\frac{1}{2}pl

If you are fortunate enough to have a square pyramid with a base length, b, and slant height, l, its formula is:

SA={b}^{2}+2\left(b\right)\left(l\right)

For spheres, calculate the surface area if you know the radius, r:

SA=4\pi {r}^{2}

For hemispheres, you calculate the surface area as the sum of the base surface area, $\pi {r}^{2}$ , plus the half sphere, $2\pi {r}^{2}$ , which gives:

SA=4\pi {r}^{2}

For cones with slant height, l, and radius, r, surface area is calculated using this formula:

SA=\pi {r}^{2}+\pi rl

If you do not know the slant height but know height, h, and radius, r, you can calculate slant height, l, using the Pythagorean Theorem:

{l}^{2}={h}^{2}+{r}^{2}

For cylinders with height, h, and radius, r, the formula for surface area is:

SA=2\pi {r}^{2}+2\pi rh