The AAS (Angle-Angle-Side) Theorem
AAS (Angle-Angle-Side) theorem
The AAS Theorem says that if two angles and the non-included side of one triangle are congruent to the corresponding parts of another triangle, the triangles are congruent.
Notice how it says "non-included side," meaning you take two consecutive angles and then move on to the next side (in either direction). You do not take the side between those two angles! (If you did, you would be using the ASA Postulate).
Mathematics is a pure science, so you are almost never stopped on the street and challenged to test two triangles for congruence. If you were, though, you could test triangles for congruence in five ways. Knowing as many methods as possible helps you, giving you flexibility to deal with any situation, whether you are stopped on the street or stumped in the classroom. This method is the Angle Angle Side, or AAS Theorem.
Proving congruent triangles
Five methods exist for testing congruence in triangles, though one is restricted for use with right triangles. Here are all five:
Side Side Side (SSS)
Side Angle Side (SAS)
Angle Side Angle - (ASA)
Hypotenuse Leg - (HL) This one is reserved for right triangles.
Angle Angle Side - (AAS)
In other lessons we have illustrated the other methods, and no, we did not just randomly rearrange "Angle" and "Side" in as many ways as we could think of. Notice, for instance, you cannot find Angle Angle Angle as a congruence proof (that is reserved for similarity), nor can you cook up a Side Side Angle postulate.
Whichever term you see sandwiched between the others, that part is included. An included angle or side is physically between the others in the triangle. So Side Angle Side (SAS) means one side, the angle next to that side, and then the side next to that angle. That side is out there, all alone, not between the angles.
For every testing method, you are checking the three parts identified between the two triangles. If corresponding parts are congruent for those three parts, the two triangles are congruent. These testing methods or proofs allow you to establish congruence by checking only half the parts (from three possible sides and three possible angles).
AAS theorem
Your textbook probably calls this a theorem, or it may be labeled a postulate; don't worry about it! Keep the concept, not the fussy words, in mind as you attempt to prove triangles congruent.
To demonstrate with actual triangles, below we proudly present △GUM and △RED.

Are they congruent? Notice the little hatch marks that indicate all the congruencies, which in mathematical shorthand uses the symbol ≅.
The congruent parts are:
∠G≅∠R
∠M≅∠D
Side GU ≅ Side RE
We know from these triangles that two interior angles are congruent (and consecutive, or next to each other), but we know nothing about the side between them. Instead, seemingly unhelpfully, we learn that another side is congruent.
Going through our toolbox full of triangle congruence testing methods, we can try each:
Side Side Side (SSS) - That won't work, because we do not know about all three sides
Side Angle Side (SAS) - That won't work, either, because we know two angles, not two sides
Angle Side Angle (ASA) - This at first looks promising, but the side we know about is not an included side; it is sticking out there, past one of the two known angles
Hypotenuse Leg (HL) - Forget about it! This is reserved for right triangles, which we don't have
Angle Angle Side (AAS) - That's the ticket! This is the one (the only one) that we can use!

Why does the AAS theorem work?
Quick, what do the interior angles of all triangles add up to?
We hope you said 180°, because that is the correct answer. If you know two angles of a triangle, then you know three angles of a triangle. That is not magic; it's mathematics:
Solving for ∠U now gives you two angles with an included side. Did you see that? We did an end run around that side that was just sticking out there, all alone, and put it between two identified angles, ∠G and ∠U. So, where once we had AAS, we scooted around the triangle and turned it into ASA, which is already a postulate.
If two angles and their included side of one triangle are all congruent to two corresponding angles and their included side of another triangle, the two triangles are congruent.
AAS theorem example
Here we offer two new triangles, △LEG and △ARM. Notice all the little hatch marks indicating congruent angles and sides:
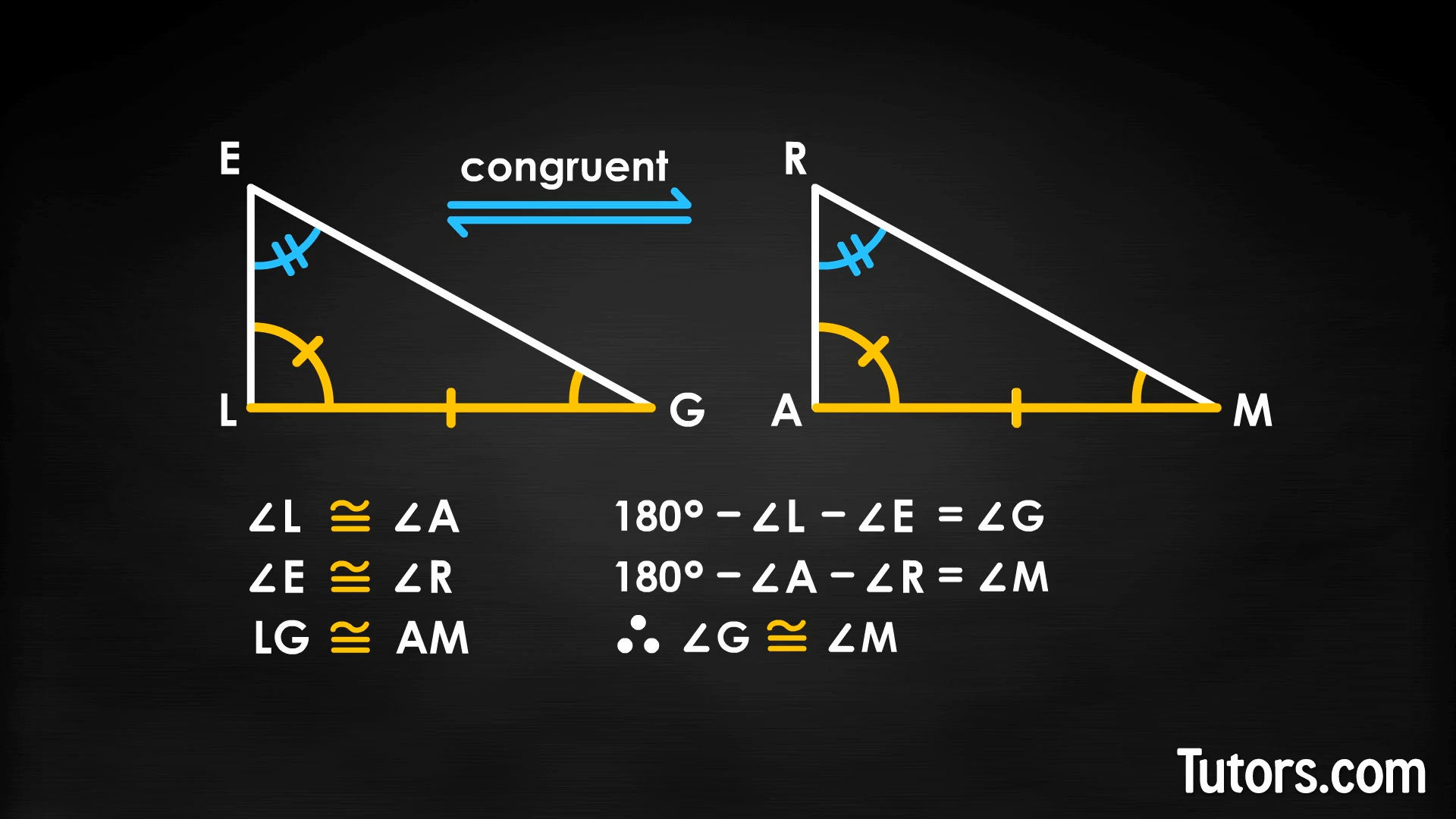
∠L ≅ ∠A
∠E ≅ ∠R
Side LG ≅ Side AM
Knowing the interior angles are congruent as listed, what else do you know?
We hope you said that ∠G ≅ ∠M, because:
180°−∠L−∠E = ∠G
180°−∠A−∠R = ∠M
∠G ≅ ∠M
What does that allow you to do now? Deploy ASA and declare the two triangles congruent, since:
∠L ≅ ∠A
Side LG ≅ Side AM
∠G ≅ ∠M
What real geometricians do
You have no need of proving the third angle's congruence and then deploying ASA, since we have, ready and waiting, the AAS Theorem. So real mathematicians and geometricians just leap right to AAS and declare the two triangles congruent.
If you have to explain this theorem to another student, friend, or random stranger on the street, you cannot make the leap from two angles to the mysterious third angle without some explanation. Then you may need to explain how we are essentially giving up on one of our original angles in favor of the third angle.
It is that mental shift, from a given angle to the newly identified third angle, that allows you to tap the awesome power of ASA and gather our previously outlying side into the proof.
Finally, after walking your pal through those steps, hit 'em with the efficiency and even more awesome power of AAS, where any two angles and a non-included side can be used to identify congruence between triangles. Pretty impressive, isn't it?
Lesson summary
Now that you have tinkered with triangles and studied these notes, you are able to recall and apply the Angle Angle Side (AAS) Theorem, know the right times to to apply AAS, make the connection between AAS and ASA, and (perhaps most helpful of all) explain to someone else how AAS helps to determine congruence in triangles.